Teaching differentiated math in a classroom with varying student need and ability is no small feat. So we asked our amazing teachers on our Facebook group, the WeAreTeachers Helpline, what hurdles and issues they’ve faced in their differentiated classrooms with the hope of finding them (and you!) real, practical math-classroom solutions.
Renowned author, educator, and Mathletics contributor Dr. Marian Small is the author of Good Questions: Great Ways to Differentiate Mathematics Instruction and More Good Questions: Great Ways to Differentiate Secondary Mathematics Instructions. Clearly, she’s ready to answer the call!
Teacher Question: I like to use online learning tools and assessments based on needs. This way I can assign something similar maybe a grade below or ahead if they are higher. But the second my back is turned, students will watch YouTube. What’s a better alternative?
Dr. Small: Although there may be value in meeting individual needs with personalized online instruction, most of us learn more effectively with others. So it might make more sense for students to work in small groups to discuss math ideas rather than work online on rich problems to learn new ideas. I like this task from Mathletics for grades 3–5. Many questions are provided that students can discuss, for example:
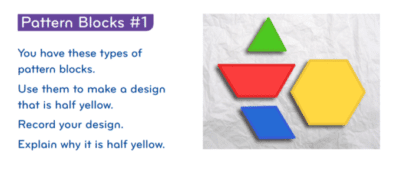
- Did your design have a line of symmetry? Did it have to have one in order to be correct?
- What color combinations are possible?
- What is the fewest number of blocks possible? Why that number?
- Why might someone who uses one yellow and two reds be correct? In what way is it not one-half yellow? Why might someone who uses one yellow and one green be correct? In what way is it not one-half yellow?
- If you duplicated your design and put the two copies together, what fraction would be yellow? Why?
Another option is reengaging online tasks that will keep students focused on the math. You might consider games such as Multiverse, which is part of Mathletics. You can try it for free.
Teacher Question: At the high school level, with high-stakes testing, differentiation is so hard, especially when you have low performers, due to lack of care and lack of prerequisite knowledge, and students who should be in the advanced class but decided not to in high school because they were taking AP classes. What are some solutions in this age bracket, who is under a lot of pressure?
Dr. Small: Differentiated math becomes easier when using open questions; it should work for low readiness to very capable students. The issue of lack of concern on the part of some students, of course, is quite different. There are no easy answers to this other than building a strong personal relationship with the student as well as ensuring they meet success and don’t give up due to failure.
Pressure is often a matter of perception or self-imposed. Getting students to deeply understand some ideas rather than glossing over too many separate small topics may actually better prepare them for testing.
Teacher Question: I have a class of 19 that are a wide range of abilities. I will have some kids do algebra work a grade ahead and some in pre-algebra. What’s my best math instruction solution, groups or independent work?
Dr. Small: Almost every teacher I meet, even in the early grades, has students who vary a lot in their abilities and readiness. I think that a huge issue that needs to change is the way we view math as a set of discrete skills that are grade-level specific instead of as a set of bigger ideas that apply to students working at a lot of levels. We can effectively address all those various students at the same time by using more open-ended questions focused on those bigger ideas; the students lagging behind the others actually learn, when responses are discussed, from their more advanced peers.
Consider this big idea in algebra: The same algebraic expression or equation can be related to different real-world situations, and different algebraic expressions or equations can describe the same real-world situation. I might give students a choice of equations and ask them to describe a real-world situation that would be described by that equation:
4x = 100 4x + 8 = 58 34x – 2 = 4x + 18 4x = 3y
Students discuss why the situation they describe makes sense, they discuss what each letter, number, or operation sign means in terms of the situation, and they discuss whether there are lots of solutions—or not.
So one student might choose the first equation and write something like: How much did it cost to buy a computer cable if four of them cost $100? The four tells how many cables, the x tells how much each costs, and the 100 tells how much four cost.
Another might choose the second one and write something like: There were a lot of cars with four people inside and a van holding eight people. Altogether, there were 58 people being transported. How many cars were there? The four tells how many in a car; the x tells how many cars there were. The eight tells how many were in the van. The 58 tells how many people altogether; the plus means to put all the groups together.
A student might choose the third one and write: Jan poured water into a 34-gallon container to a certain level. She then dumped out two gallons of water. With the water that was left, she was able to fill an 18-gallon container and a bunch of four-gallon containers to the same level as the 34-gallon container. How full did she fill the 34-gallon and 4-gallon containers?
The 34 tells that one container held 34 gallons. The x tells the fraction of the container that was full. The minus sign says that some water was poured out, and the two tells how much was poured out. The four tells that some containers held four gallons, and the x tells how filled they were. The plus tells that there was some more water, and the 18 tells how many more gallons.
A student might choose the fourth one and write: Jane had four identical bags of flour that had the same mass as Lisa’s three identical bags. How much flour could have been in each of Jane’s bags and Lisa’s bags? The four tells how many bags Jane had, and the three tells how many Lisa had. The x tells the mass of Jane’s bag, and the y tells the mass of Lisa’s bag.
Teacher Question: How do you reach the lowest achieving accelerated students while pushing forward the content?
Dr. Small: Try using open questions. More open-ended questions allow access to all students but stretch strong students. They work at any level. Here are a few examples:
Early elementary: A number is represented using 12 base ten blocks. List lots of possible numbers, some big and some small. What do you notice about all the numbers you found?
Later elementary: You divide a large number by a small one and the result is between 20 and 30; there is a remainder of one. What could you have divided?
Middle school: A composite shape that includes some parallelograms and some triangles has a total area of 40 square inches. What could the dimensions of each part of the shape be?
High school: Choose a function f(x). Figure out each of these. Tell how they are alike and different.
f(x+3) f(x – 3) f(3x) 3f(x)
Mathletics incorporates a number of open-ended tasks, like the one mentioned above, but for every K–8 grade and for a number of strands. Check out this one involving predicting area on a grid based on the number of grid dots on its border.
Want to try open-ended tasks in your classroom? Sign up for a free Mathletics trial to get two virtual interactives today.

