40 Inspiring Poetry Games and Activities for Kids and Teens

27+ Best Autism Resources for Educators

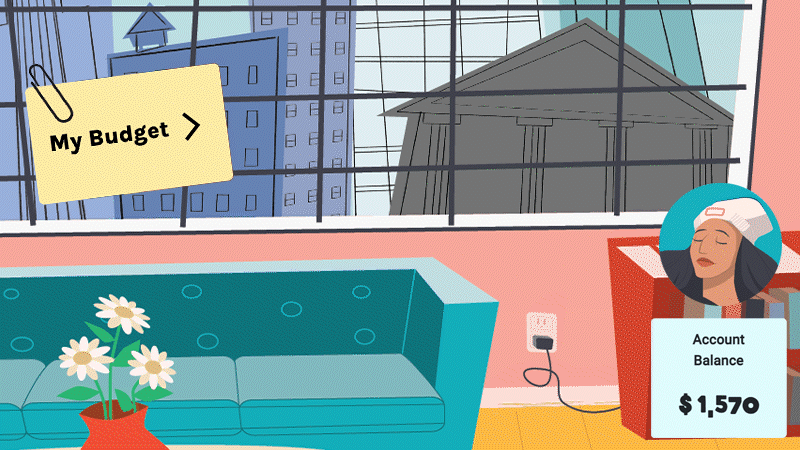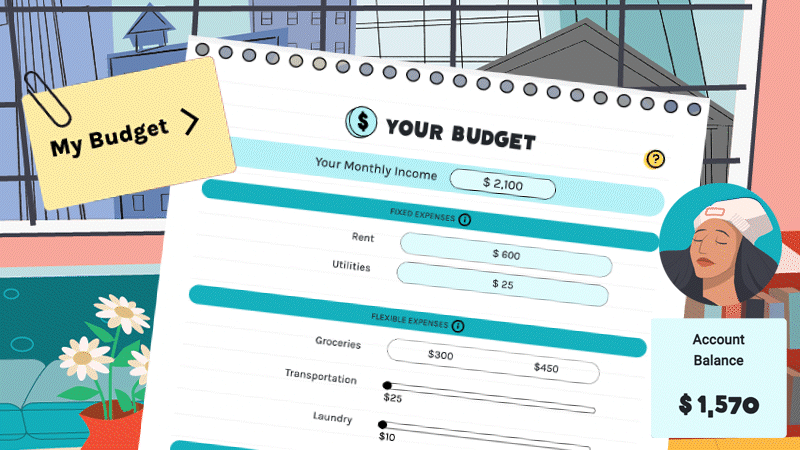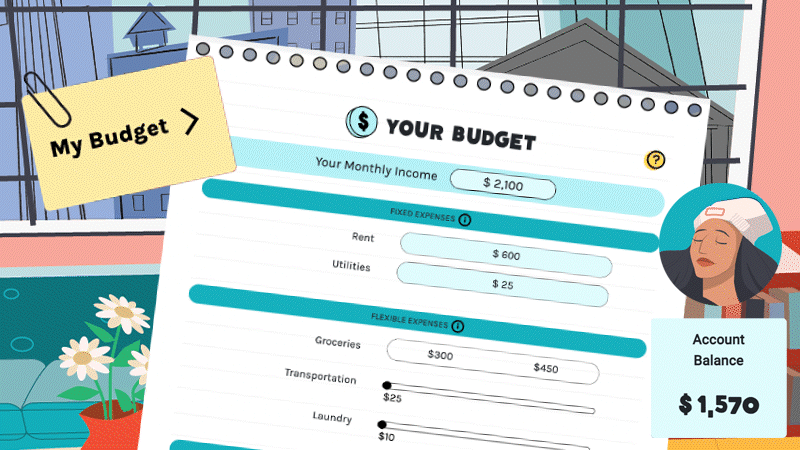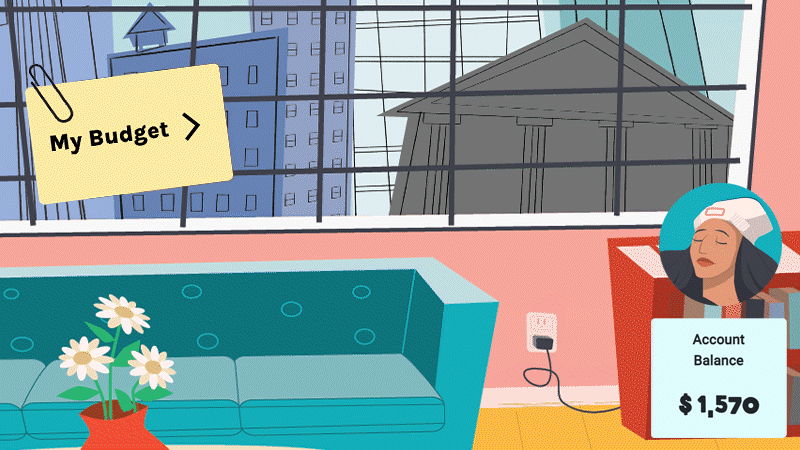
Financial Literacy for Kids: Lessons, Activities, and Teaching Tips
Top Stories

Get Our Free Spring Posters & Bring a Spot of Sunshine to Your Classroom
Hope, growth, and happiness.
Classroom Ideas
Browse by Topic
Discover classroom ideas, teaching strategies, and actionable tips for the subjects you teach every day.
Reading
Our top teacher-approved book lists, reading tips, and so much more!
Math
Find tips, resources, lesson plans, and games for K-12 that make math teachers’ lives easier.
Science
Science experiments, lesson plans, science themed books, and more!
Arts
Find everything from creative ideas for using music and theater at school to craft projects you’ll love.
ESL
Tips for English as a Second Language and English Language Learner teachers.
Social Studies
Economics, history, geography, sociology, anthropology, psychology … we have you covered.